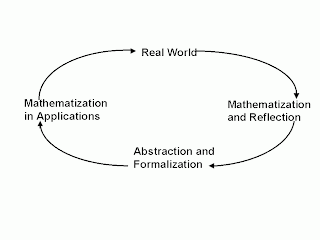
PMRI’s
journey in Surabaya
Researcher : Siti M.Amin, Gatot Sulanjono
Translation
and adaptation by Annelies Gijse
Introduction
In July 2001, the staff meeting in the Department of
Mathematics of University of Surabaya (UNESA), Professor R. Soedjadi announced
an important project that we were going to involved with. He explained that a
group of mathematicians including him, had committed themselves to innovating
the quality of the existing mathematics educational system of Indonesia
especially in primary school. They introduced Realistic Mathematics Education
(RME) principle, they believed that this mathematics approach was suitable to the
situation in Indonesia mainly to students in primary school. To realize the
noble cause, Mrs. Siti Khabibah was invited to join the team. The team start
with three primary school in Surabaya, that is Jambangan Islamic School, Unesa
Lab School, and Al Hikmah Primary School. They choose Islamic primary school
because they want to change their style in mathematics instruction, because
they thought
that public Islamic primary school have different learning methods,
specifically in mathematics,
For the purpose of the experiment, the team was ask to decide
upon particular topic for each school level (grade 1, 2 and 3)which would be
experiment within limited measures. They choose topics : non- standard
measurement (inch, feet, etc)for grade 1, non-standard measurement (stick,
beads) for grade 2, and fraction for grade 3. The topics for grade 1 and 2 were
composed by Siti M.Amin and for Grade 3 by Siti Khabibah, and Mr.Ilham assisted
in the development of the
experimental material.
The first
pilot
Between October until November, after an agreement had been
reached between the team from Unesa and
the representatives of affiliated schools, a pilot was conducted at the three
schools to implement the material that already had been developed. The pilot was
supervised by observers from Unesa in each class. They were observing the
learning process in the classrooms.
They observed some interesting facts during the learning
process. For example, usually the students of grade 3 always wanted to check
whether they had found the right solution or approach to open-end equations
after they had solved the problem, whereas his behaviour was not found among students of grade 1 and
2. During the lesson, they found that most of students enthusiastic and happy
during lesson. The students
performed excellently in stating their own opinion or presented their ways when
solving the problems. But on the other
hands, teacher impatient to wait the students initiative or answers and also in
posing question to guide students with.
After learning process in the class, they made the reflection
session for every lesson. The teachers generally admitted that they were
impatient to wait the students to come up with initiatives to solve the
problems using their own method. The teachers of grade 3 classes presumed that
their students kept on asking which method or answer was
right because they have been conditioned to solve problems with the one single
method or solution that was provided by teacher. But now the teachers had
started to tell the students to use
other method to solve their problems, however the students were still confused.
In the reflection session discussed about issues such as the
presentation of probing questions, class management matters, and comprehensive
study of materials, also teacher shared their experience in the lesson process.
In general, teachers appeared to rather good at class management but they
needed improvement on respecting the different opinions of the students an
their different ways of thinking and they certainly needed some more patience
too.
Further
experimentation and implementation
At the First National Wokshop
in August 2001 in Bandung , the RME team decided that the program being
experimented required a name. The team agreed on the name “ Pendidikan
Matematika Realistik Indonesia (PMRI)”. The core team conclude that each
Teacher Education College was subject to implement the experiment in
comprehensive manner and decided that the experiment was to be performed in
phases, beginning from grade 1 classes.
The comprehensive experiment
for grade 1 started in 2002-2003, it was conducted one single class at each
affiliated school of the teacher education college, and the experiment
performed on the grade 1classes was subsequently followed by implementation of
the program into grade 1 classes in following school year 0f 2003 – 2004. While
grade 1 conducted PMRI in implementation phase, students of grade 2 began their
experimental phase. This cycle continued, in which experimentation and
implementation were applied continuously.
Unesa had already composed
instruction material for Semester 1 of grade 1 in 2001, grade 3 in 2003 and in
2007 we received the task to composed instruction material for grade 5. In 2007
was when the team begun to understand how to write proper PMRI material,
because was not easy to make context and the team needed to focus not only on
mathematical progress but on developing the students thinking too. So, 2007 was
momentous in that the team were finally able to make proper materials.
Result
The team found that the
teachers experienced the unexpected outcome from their students during the
lesson they were happy to teach according to the PMRI approach. Most of
students were able to state how they had discovered methods to solve problems
appropriately, and they were able to communicate their ideas clearly. The
teachers expressed that PMRI has made teaching more enjoyable because found
unexpected abilities in their students which they had never seen before.
By : Wuli Oktiningrum